Physics For Scientists And Engineers 6E - part 162

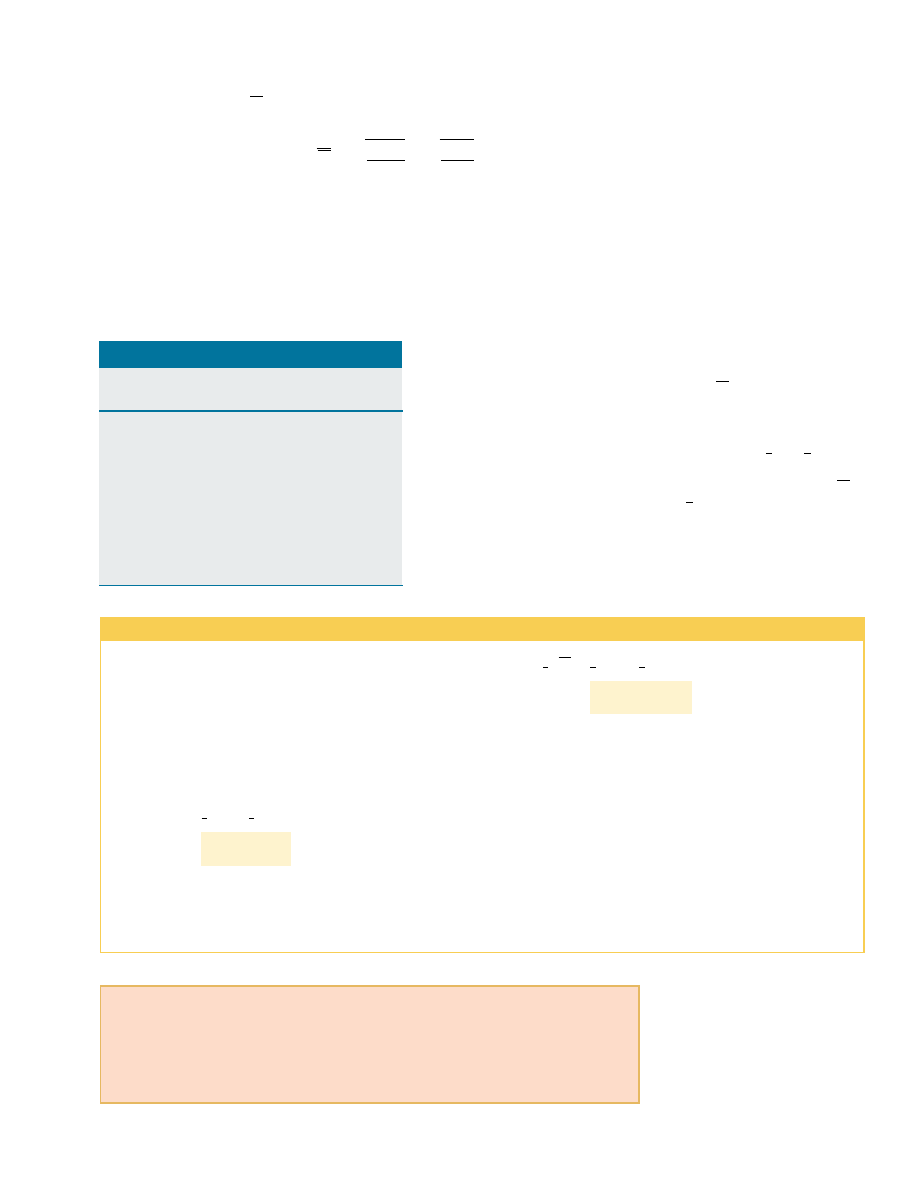
The  square  root  of 

is  called  the  root-mean-square (rms)  speed of  the  molecules.

From Equation 21.4 we find that the rms speed is

(21.7)

where M is the molar mass in kilograms per mole and is equal to mN

A

. This expression

shows that, at a given temperature, lighter molecules move faster, on the average, than
do  heavier  molecules.  For  example,  at  a  given  temperature,  hydrogen  molecules,
whose  molar  mass  is  2.02 % 10

!

3

kg/mol,  have  an  average  speed  approximately  four

times that of oxygen molecules, whose molar mass is 32.0 % 10

!

3

kg/mol. Table 21.1

lists the rms speeds for various molecules at 20°C.

v

rms

#

√

v

2

#

√

3k

B

T

m

#

√

3RT

M

v

2

SECTION 21.1 •  Molecular Model of an Ideal Gas

645

Root-mean-square speed

▲

PITFALL PREVENTION

21.1 The Square Root of

the Square?

Notice  that  taking  the  square
root  of 

does  not  “undo”  the

square because we have taken an
average between squaring and tak-
ing  the  square  root.  While  the
square  root  of 

is  because

the squaring is done after the av-
eraging,  the  square  root  of 

is

not , but rather v

rms

.

v

v

2

v

(v)

2

v

2

Molar mass

v

rms

Gas

(g/mol)

at 20!C(m/s)

H

2

2.02

1 902

He

4.00

1 352

H

2

O

18.0

637

Ne

20.2

602

N

2

or CO

28.0

511

NO

30.0

494

O

2

32.0

478

CO

2

44.0

408

SO

2

64.1

338

Table 21.1

Some rms Speeds

Example 21.1 A Tank of Helium

A  tank  used  for  filling  helium  balloons  has  a  volume  of
0.300 m

3

and  contains  2.00 mol  of  helium  gas  at  20.0°C.

Assume that the helium behaves like an ideal gas.

(A)

What is the total translational kinetic energy of the gas

molecules?

Solution Using  Equation  21.6  with  n # 2.00 mol  and  T #
293 K, we find that

(B)

What is the average kinetic energy per molecule?

Solution Using  Equation  21.4,  we  find  that  the  average
kinetic energy per molecule is

7.30 % 10

3

 J

#

K

tot

  

trans

#

3

2

nRT #

3

2

(2.00 mol)(8.31 J/mol&K)(293 K)

What  If?

What  if  the  temperature  is  raised  from  20.0°C  to

40.0°C?  Because  40.0  is  twice  as  large  as  20.0,  is  the  total
translational  energy  of  the  molecules  of  the  gas  twice  as
large at the higher temperature?

Answer The  expression  for  the  total  translational  energy
depends on the temperature, and the value for the tempera-
ture  must  be  expressed  in  kelvins,  not  in  degrees  Celsius.
Thus,  the  ratio  of  40.0  to  20.0  is not the  appropriate  ratio.
Converting  the  Celsius  temperatures  to  kelvins,  20.0°C  is
293 K  and  40.0°C  is  313 K.  Thus,  the  total  translational
energy increases by a factor of 313 K/293 K # 1.07.

6.07 % 10

!

21 

J

#

1

2

mv

2

#

3

2

k

B

T #

 

3

2

(1.38 % 10

!

23

 J/K)(293 K)

Quick Quiz 21.1

Two containers hold an ideal gas at the same temperature

and pressure. Both containers hold the same type of gas but container B has twice the
volume of container A. The average translational kinetic energy per molecule in con-
tainer B is (a) twice that for container A (b) the same as that for container A (c) half
that for container A (d) impossible to determine.

646

CHAPTE R 21 •  The Kinetic Theory of Gases

21.2 Molar Specific Heat of an Ideal Gas

Consider an ideal gas undergoing several processes such that the change in tempera-
ture is "T # T

f

!

T

i

for all processes. The temperature change can be achieved by tak-

ing a variety of paths from one isotherm to another, as shown in Figure 21.3. Because
"

T is the same for each path, the change in internal energy "E

int

is the same for all

paths. However, we know from the first law, Q # "E

int

!

W, that the heat Q is different

for  each  path  because  W (the  negative  of  the  area  under  the  curves)  is  different  for
each path. Thus, the heat associated with a given change in temperature does not have
a unique value.

We can address this difficulty by defining specific heats for two processes that fre-

quently occur: changes at constant volume and changes at constant pressure. Because
the  number  of  moles  is  a  convenient  measure  of  the  amount  of  gas,  we  define  the
molar specific heats associated with these processes with the following equations:

(21.8)

(21.9)

where C

V

is the 

molar specific heat at constant volume and C

P

is the 

molar specific

heat at constant pressure. When we add energy to a gas by heat at constant pressure,
not only does the internal energy of the gas increase, but work is done on the gas be-
cause of the change in volume. Therefore, the heat Q

constant P

must account for both

the increase in internal energy and the transfer of energy out of the system by work.
For this reason, Q

constant P

is greater than Q

constant V

for given values of n and "T. Thus,

C

P

is greater than C

V

.

In the previous section, we found that the temperature of a gas is a measure of the

average translational kinetic energy of the gas molecules. This kinetic energy is associ-
ated with the motion of the center of mass of each molecule. It does not include the
energy associated with the internal motion of the molecule—namely, vibrations and ro-
tations  about  the  center  of  mass.  This  should  not  be  surprising  because  the  simple
kinetic theory model assumes a structureless molecule.

In view of this, let us first consider the simplest case of an ideal monatomic gas, that

is,  a  gas  containing  one  atom  per  molecule,  such  as  helium,  neon,  or  argon.  When
energy is added to a monatomic gas in a container of fixed volume, all of the added en-
ergy  goes  into  increasing  the  translational  kinetic  energy  of  the  atoms.  There  is  no
other way to store the energy in a monatomic gas. Therefore, from Equation 21.6, we
see that the internal energy E

int

of N molecules (or n mol) of an ideal monatomic gas is

(21.10)

Note  that  for  a  monatomic  ideal  gas,  E

int

is  a  function  of  T only,  and  the  functional

relationship is given by Equation 21.10. In general, the internal energy of an ideal gas
is a function of T only, and the exact relationship depends on the type of gas.

E

int

#

K

tot

  

trans

#

3

2

Nk

B

T #

3

2

nRT

Q # nC

P

 "T

   

(constant pressure)

Q # nC

V

 "T

   

(constant volume)

P

V

Isotherms

i

f

f

 ′

T + 

∆T

f

 ′′

T

Figure 21.3 An ideal gas is taken

from one isotherm at temperature

T to another at temperature

T $ "T along three different 

paths.

Internal energy of an ideal

monatomic gas

Quick  Quiz  21.2

Consider  again  the  situation  in  Quick  Quiz  21.1.  The

internal energy of the gas in container B is (a) twice that for container A (b) the same
as that for container A (c) half that for container A (d) impossible to determine.

Quick Quiz 21.3

Consider again the situation in Quick Quiz 21.1. The rms

speed of the gas molecules in container B is (a) twice that for container A (b) the same
as that for container A (c) half that for container A (d) impossible to determine.

If energy is transferred by heat to a system at constant volume, then no work is done

on  the  system.  That  is, 

for  a  constant-volume  process.  Hence,  from

the first law of thermodynamics, we see that

(21.11)

In other words, all of the energy transferred by heat goes into increasing the internal
energy of the system. A constant-volume process from i to f for an ideal gas is described
in Figure 21.4, where "T is the temperature difference between the two isotherms. Sub-
stituting the expression for Q given by Equation 21.8 into Equation 21.11, we obtain

(21.12)

If the molar specific heat is constant, we can express the internal energy of a gas as

E

int

#

nC

V

T

This equation applies to all ideal gases—to gases having more than one atom per mole-
cule as well as to monatomic ideal gases. In the limit of infinitesimal changes, we can
use Equation 21.12 to express the molar specific heat at constant volume as

(21.13)

Let us now apply the results of this discussion to the monatomic gas that we have

been  studying.  Substituting  the  internal  energy  from  Equation  21.10  into  Equation
21.13, we find that

(21.14)

This expression predicts a value of 

for all monatomic gases.

This prediction is in excellent agreement with measured values of molar specific heats
for such gases as helium, neon, argon, and xenon over a wide range of temperatures
(Table 21.2). Small variations in Table 21.2 from the predicted values are due to the

C

V

#

3

2

R # 12.5 J/mol&K

C

V

#

3

2

R

C

V

#

1
n

  

dE

int

dT

"

E

int

#

nC

V

 

 "T

Q # "E

int

W # !$P

 

dV # 0

SECTION 21.2 •  Molar Specific Heat of an Ideal Gas

647

Active Figure 21.4 Energy is trans-

ferred by heat to an ideal gas in two

ways. For the constant-volume path

i : f, all the energy goes into in-

creasing the internal energy of the

gas because no work is done. Along

the constant-pressure path i : f ',

part of the energy transferred in by

heat is transferred out by work.

At the Active Figures link

at http://www.pse6.com, you

can choose initial and final

temperatures for one mole of

an ideal gas undergoing

constant-volume and constant

pressure processes and mea-

sure Q, W, "E

int

, C

V

, and C

P

.

P

V

T + 

∆T

T

i

f

f

 ′

Isotherms

Molar Specific Heat ( J/mol " K)

a

Gas

C

P

C

V

C

P

#

C

V

$

%

C

P

/C

V

Monatomic Gases

He

20.8

12.5

8.33

1.67

Ar

20.8

12.5

8.33

1.67

Ne

20.8

12.7

8.12

1.64

Kr

20.8

12.3

8.49

1.69

Diatomic Gases

H

2

28.8

20.4

8.33

1.41

N

2

29.1

20.8

8.33

1.40

O

2

29.4

21.1

8.33

1.40

CO

29.3

21.0

8.33

1.40

Cl

2

34.7

25.7

8.96

1.35

Polyatomic Gases

CO

2

37.0

28.5

8.50

1.30

SO

2

40.4

31.4

9.00

1.29

H

2

O

35.4

27.0

8.37

1.30

CH

4

35.5

27.1

8.41

1.31

Molar Specific Heats of Various Gases

Table 21.2

a

All values except that for water were obtained at 300 K.

fact that real gases are not ideal gases. In real gases, weak intermolecular interactions
occur, which are not addressed in our ideal gas model.

Now suppose that the gas is taken along the constant-pressure path i : f ' shown in

Figure 21.4. Along this path, the temperature again increases by "T. The energy that
must be transferred by heat to the gas in this process is Q # nC

P

"

T. Because the vol-

ume changes in this process, the work done on the gas is W # ! P "V where P is the
constant pressure at which the process occurs. Applying the first law of thermodynam-
ics to this process, we have

(21.15)

In  this  case,  the  energy  added  to  the  gas  by  heat  is  channeled  as  follows:  Part  of  it
leaves the system by work (that is, the gas moves a piston through a displacement), and
the remainder appears as an increase in the internal energy of the gas. But the change
in internal energy for the process i : f ' is equal to that for the process i : f because
E

int

depends only on temperature for an ideal gas and because "T is the same for both

processes.  In  addition,  because  PV # nRT,  we  note  that  for  a  constant-pressure
process,  P "V # nR "T.  Substituting  this  value  for  P "V into  Equation  21.15  with
"

E

int

#

nC

V

"

T (Eq. 21.12) gives

C

P

!

C

V

#

R

(21.16)

This expression applies to any ideal gas. It predicts that the molar specific heat of an
ideal gas at constant pressure is greater than the molar specific heat at constant volume
by an amount R, the universal gas constant (which has the value 8.31 J/mol & K). This
expression is applicable to real gases, as the data in Table 21.2 show.

Because C

V

#

R for a monatomic ideal gas, Equation 21.16 predicts a value C

P

#

R # 20.8 J/mol & K for the molar specific heat of a monatomic gas at constant pressure.

The ratio of these molar specific heats is a dimensionless quantity ( (Greek gamma):

(21.17)

Theoretical values  of C

V

,  C

P

and  ( are  in excellent agreement  with experimental

values obtained for monatomic gases, but they are in serious disagreement with the val-
ues  for  the  more  complex  gases  (see  Table  21.2).  This  is  not  surprising  because  the
value C

V

#

R was derived for a monatomic ideal gas and we expect some additional

contribution to the molar specific heat from the internal structure of the more com-
plex molecules. In Section 21.4, we describe the effect of molecular structure on the
molar  specific  heat  of  a  gas.  The  internal  energy—and,  hence,  the  molar  specific
heat—of a complex gas must include contributions from the rotational and the vibra-
tional motions of the molecule.

In the case of solids and liquids heated at constant pressure, very little work is done

because  the  thermal  expansion  is  small.  Consequently,  C

P

and  C

V

are  approximately

equal for solids and liquids.

3

2

( #

C

P

C

V

#

5R/2
3R/2

#

5
3

#

1.67

5

2

3

2

nC

V

 

 

"

T # nC

P

 

 

"

T ! nR

  

"

T

"

E

int

#

Q $ W # nC

P

 

 

"

T

 

$

(!P

  

"

V

  

)

648

CHAPTE R 21 •  The Kinetic Theory of Gases

Quick Quiz 21.4

How does the internal energy of an ideal gas change as it

follows path i : f in Figure 21.4? (a) E

int

increases. (b) E

int

decreases. (c) E

int

stays the

same. (d) There is not enough information to determine how E

int

changes.

Quick Quiz 21.5

How does the internal energy of an ideal gas change as it

follows  path  f : f ' along  the  isotherm  labeled  T $ "T in  Figure  21.4?  (a)  E

int

increases. (b) E

int

decreases. (c) E

int

stays the same. (d) There is not enough informa-

tion to determine how E

int

changes.

Ratio of molar specific heats for

a monatomic ideal gas